Mathematics of Demand Billing

Some interesting math on Demand.  Average is really the arithmetic mean.  This is illustrated by:



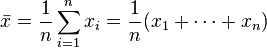
Which would be expanded to:

1/60 ( X1+ X2 + X3 ....+ X60)

Since each X is the sum of the draw of all of the appliances in the household for a minute and there are 60 of them (60 minutes in an hour) what they're actually measuring is the usage separately for every hour during the peak period for a month and keeping the highest value to be used in billing calculations. This is a rolling average covering every 60 minute period during the peak for the month.

This would mean that a test where a 1000W appliance is run for 15 minutes and nothing else was run for an hour would result in (1000 * 15) / 60 = 250 Watts.  Exactly what they are predicting will happen.  However, if a base load of 500W runs continuously and we add the 1000W appliance we will get:

((1000 * 15) + (500 * 60) ) / 60 = 750 W

This is completely supported by the data in my previous post where I did the calcs for each minute during the example hour.  By the way, my Demand reading so far this month is 1.8kW.  Been keeping it pretty lean.

This is going to be an interesting test on Friday.  I may come out with egg on my face.